Factoring Numbers on the ISEE
All Levels
ISEE Question: How many different factors does 48 have?
Solution: We’re going to solve this using the rainbow method (for a different way of doing it, see the “Prime Factors and Prime Factorization on the ISEE” lesson).
STEP 1: Write the number 1 on the left side of your work space, and the number you’re factoring—in this case, 48—on the right side. Connect them with an arc.

STEP 2: Next, consider the number 2. Since 48 is even, it is divisible by 2, so write 2 on the left side of your work space and its factor pair—24—on the right.

STEP 3: Now, consider the next number, 3. Since 48 is divisible by 3 (see divisibility rules), you would write 3 on the left and its factor pair—16—on the right. Continue this process for 4 (a factor pair with 12), 5 (not a factor, so don’t write it down), and 6 (a factor pair with 8), and 7 (not a factor). Once your factors have met in the middle of your rainbow, you can be certain you’ve found all the factors of your number.
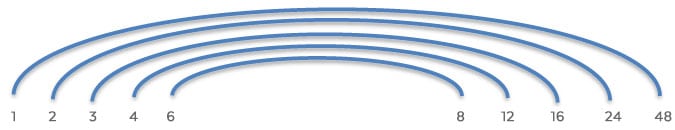
Answer: 48 has ten factors and they are 1, 2, 3, 4, 6, 8, 12, 16, 24, 48.
Helpful Tip: If a number is divisible by a given factor, it is also divisible by all of that factor’s factors. For example, since 48 is divisible by 24, we know it’s also divisible by 2, 3, 4, 6, 8, and 12.




